if your null and alternative hypothesis are

Interested in teaching this course?

Lumen can help! Review our up-to-date Introduction to Statistics by clicking the link below. From there, you can request a demo and review the course materials in your Learning Management System (LMS).

Module 9: Hypothesis Testing With One Sample

Null and alternative hypotheses, learning outcomes.

• Describe hypothesis testing in general and in practice

The actual test begins by considering two  hypotheses . They are called the null hypothesis and the alternative hypothesis . These hypotheses contain opposing viewpoints.

H 0 : The null hypothesis: It is a statement about the population that either is believed to be true or is used to put forth an argument unless it can be shown to be incorrect beyond a reasonable doubt.

H a : The alternative hypothesis : It is a claim about the population that is contradictory to H 0 and what we conclude when we reject H 0 .

Since the null and alternative hypotheses are contradictory, you must examine evidence to decide if you have enough evidence to reject the null hypothesis or not. The evidence is in the form of sample data.

After you have determined which hypothesis the sample supports, you make adecision. There are two options for a  decision . They are “reject H 0 ” if the sample information favors the alternative hypothesis or “do not reject H 0 ” or “decline to reject H 0 ” if the sample information is insufficient to reject the null hypothesis.

Mathematical Symbols Used in  H 0 and H a :

H 0 always has a symbol with an equal in it. H a never has a symbol with an equal in it. The choice of symbol depends on the wording of the hypothesis test. However, be aware that many researchers (including one of the co-authors in research work) use = in the null hypothesis, even with > or < as the symbol in the alternative hypothesis. This practice is acceptable because we only make the decision to reject or not reject the null hypothesis.

H 0 : No more than 30% of the registered voters in Santa Clara County voted in the primary election. p ≤ 30

H a : More than 30% of the registered voters in Santa Clara County voted in the primary election. p > 30

A medical trial is conducted to test whether or not a new medicine reduces cholesterol by 25%. State the null and alternative hypotheses.

H 0 : The drug reduces cholesterol by 25%. p = 0.25

H a : The drug does not reduce cholesterol by 25%. p ≠ 0.25

We want to test whether the mean GPA of students in American colleges is different from 2.0 (out of 4.0). The null and alternative hypotheses are:

H 0 : μ = 2.0

H a : μ ≠ 2.0

We want to test whether the mean height of eighth graders is 66 inches. State the null and alternative hypotheses. Fill in the correct symbol (=, ≠, ≥, <, ≤, >) for the null and alternative hypotheses. H 0 : μ __ 66 H a : μ __ 66

• H 0 : μ = 66
• H a : μ ≠ 66

We want to test if college students take less than five years to graduate from college, on the average. The null and alternative hypotheses are:

H 0 : μ ≥ 5

H a : μ < 5

We want to test if it takes fewer than 45 minutes to teach a lesson plan. State the null and alternative hypotheses. Fill in the correct symbol ( =, ≠, ≥, <, ≤, >) for the null and alternative hypotheses. H 0 : μ __ 45 H a : μ __ 45

• H 0 : μ ≥ 45
• H a : μ < 45

In an issue of U.S. News and World Report , an article on school standards stated that about half of all students in France, Germany, and Israel take advanced placement exams and a third pass. The same article stated that 6.6% of U.S. students take advanced placement exams and 4.4% pass. Test if the percentage of U.S. students who take advanced placement exams is more than 6.6%. State the null and alternative hypotheses.

H 0 : p ≤ 0.066

H a : p > 0.066

On a state driver’s test, about 40% pass the test on the first try. We want to test if more than 40% pass on the first try. Fill in the correct symbol (=, ≠, ≥, <, ≤, >) for the null and alternative hypotheses. H 0 : p __ 0.40 H a : p __ 0.40

• H 0 : p = 0.40
• H a : p > 0.40

Concept Review

In a  hypothesis test , sample data is evaluated in order to arrive at a decision about some type of claim. If certain conditions about the sample are satisfied, then the claim can be evaluated for a population. In a hypothesis test, we: Evaluate the null hypothesis , typically denoted with H 0 . The null is not rejected unless the hypothesis test shows otherwise. The null statement must always contain some form of equality (=, ≤ or ≥) Always write the alternative hypothesis , typically denoted with H a or H 1 , using less than, greater than, or not equals symbols, i.e., (≠, >, or <). If we reject the null hypothesis, then we can assume there is enough evidence to support the alternative hypothesis. Never state that a claim is proven true or false. Keep in mind the underlying fact that hypothesis testing is based on probability laws; therefore, we can talk only in terms of non-absolute certainties.

Formula Review

H 0 and H a are contradictory.

Candela Citations

• OpenStax, Statistics, Null and Alternative Hypotheses. Provided by : OpenStax. Located at : http://cnx.org/contents/[email protected]:58/Introductory_Statistics . License : CC BY: Attribution
• Introductory Statistics . Authored by : Barbara Illowski, Susan Dean. Provided by : Open Stax. Located at : http://cnx.org/contents/[email protected] . License : CC BY: Attribution . License Terms : Download for free at http://cnx.org/contents/[email protected]
• Simple hypothesis testing | Probability and Statistics | Khan Academy. Authored by : Khan Academy. Located at : https://youtu.be/5D1gV37bKXY . License : All Rights Reserved . License Terms : Standard YouTube License

Have a thesis expert improve your writing

Check your thesis for plagiarism in 10 minutes, generate your apa citations for free.

• Knowledge Base
• Null and Alternative Hypotheses | Definitions & Examples

Null and Alternative Hypotheses | Definitions & Examples

Published on 5 October 2022 by Shaun Turney . Revised on 6 December 2022.

The null and alternative hypotheses are two competing claims that researchers weigh evidence for and against using a statistical test :

• Null hypothesis (H 0 ): There’s no effect in the population .
• Alternative hypothesis (H A ): There’s an effect in the population.

The effect is usually the effect of the independent variable on the dependent variable .

Table of contents

Answering your research question with hypotheses, what is a null hypothesis, what is an alternative hypothesis, differences between null and alternative hypotheses, how to write null and alternative hypotheses, frequently asked questions about null and alternative hypotheses.

The null and alternative hypotheses offer competing answers to your research question . When the research question asks “Does the independent variable affect the dependent variable?”, the null hypothesis (H 0 ) answers “No, there’s no effect in the population.” On the other hand, the alternative hypothesis (H A ) answers “Yes, there is an effect in the population.”

The null and alternative are always claims about the population. That’s because the goal of hypothesis testing is to make inferences about a population based on a sample . Often, we infer whether there’s an effect in the population by looking at differences between groups or relationships between variables in the sample.

You can use a statistical test to decide whether the evidence favors the null or alternative hypothesis. Each type of statistical test comes with a specific way of phrasing the null and alternative hypothesis. However, the hypotheses can also be phrased in a general way that applies to any test.

The null hypothesis is the claim that there’s no effect in the population.

If the sample provides enough evidence against the claim that there’s no effect in the population ( p ≤ α), then we can reject the null hypothesis . Otherwise, we fail to reject the null hypothesis.

Although “fail to reject” may sound awkward, it’s the only wording that statisticians accept. Be careful not to say you “prove” or “accept” the null hypothesis.

Null hypotheses often include phrases such as “no effect”, “no difference”, or “no relationship”. When written in mathematical terms, they always include an equality (usually =, but sometimes ≥ or ≤).

Examples of null hypotheses

The table below gives examples of research questions and null hypotheses. There’s always more than one way to answer a research question, but these null hypotheses can help you get started.

*Note that some researchers prefer to always write the null hypothesis in terms of “no effect” and “=”. It would be fine to say that daily meditation has no effect on the incidence of depression and p 1 = p 2 .

The alternative hypothesis (H A ) is the other answer to your research question . It claims that there’s an effect in the population.

Often, your alternative hypothesis is the same as your research hypothesis. In other words, it’s the claim that you expect or hope will be true.

The alternative hypothesis is the complement to the null hypothesis. Null and alternative hypotheses are exhaustive, meaning that together they cover every possible outcome. They are also mutually exclusive, meaning that only one can be true at a time.

Alternative hypotheses often include phrases such as “an effect”, “a difference”, or “a relationship”. When alternative hypotheses are written in mathematical terms, they always include an inequality (usually ≠, but sometimes > or <). As with null hypotheses, there are many acceptable ways to phrase an alternative hypothesis.

Examples of alternative hypotheses

The table below gives examples of research questions and alternative hypotheses to help you get started with formulating your own.

Null and alternative hypotheses are similar in some ways:

• They’re both answers to the research question
• They both make claims about the population
• They’re both evaluated by statistical tests.

However, there are important differences between the two types of hypotheses, summarized in the following table.

To help you write your hypotheses, you can use the template sentences below. If you know which statistical test you’re going to use, you can use the test-specific template sentences. Otherwise, you can use the general template sentences.

The only thing you need to know to use these general template sentences are your dependent and independent variables. To write your research question, null hypothesis, and alternative hypothesis, fill in the following sentences with your variables:

Does independent variable affect dependent variable ?

• Null hypothesis (H 0 ): Independent variable does not affect dependent variable .
• Alternative hypothesis (H A ): Independent variable affects dependent variable .

Test-specific

Once you know the statistical test you’ll be using, you can write your hypotheses in a more precise and mathematical way specific to the test you chose. The table below provides template sentences for common statistical tests.

Note: The template sentences above assume that you’re performing one-tailed tests . One-tailed tests are appropriate for most studies.

The null hypothesis is often abbreviated as H 0 . When the null hypothesis is written using mathematical symbols, it always includes an equality symbol (usually =, but sometimes ≥ or ≤).

The alternative hypothesis is often abbreviated as H a or H 1 . When the alternative hypothesis is written using mathematical symbols, it always includes an inequality symbol (usually ≠, but sometimes < or >).

A research hypothesis is your proposed answer to your research question. The research hypothesis usually includes an explanation (‘ x affects y because …’).

A statistical hypothesis, on the other hand, is a mathematical statement about a population parameter. Statistical hypotheses always come in pairs: the null and alternative hypotheses. In a well-designed study , the statistical hypotheses correspond logically to the research hypothesis.

Cite this Scribbr article

If you want to cite this source, you can copy and paste the citation or click the ‘Cite this Scribbr article’ button to automatically add the citation to our free Reference Generator.

Turney, S. (2022, December 06). Null and Alternative Hypotheses | Definitions & Examples. Scribbr. Retrieved 9 December 2024, from https://www.scribbr.co.uk/stats/null-and-alternative-hypothesis/

Is this article helpful?

Shaun Turney

Other students also liked, levels of measurement: nominal, ordinal, interval, ratio, the standard normal distribution | calculator, examples & uses, types of variables in research | definitions & examples.

• Key Differences

Know the Differences & Comparisons

Difference Between Null and Alternative Hypothesis

Null hypothesis implies a statement that expects no difference or effect. On the contrary, an alternative hypothesis is one that expects some difference or effect. Null hypothesis This article excerpt shed light on the fundamental differences between null and alternative hypothesis.

Content: Null Hypothesis Vs Alternative Hypothesis

Comparison chart, definition of null hypothesis.

A null hypothesis is a statistical hypothesis in which there is no significant difference exist between the set of variables. It is the original or default statement, with no effect, often represented by H 0 (H-zero). It is always the hypothesis that is tested. It denotes the certain value of population parameter such as µ, s, p. A null hypothesis can be rejected, but it cannot be accepted just on the basis of a single test.

Definition of Alternative Hypothesis

A statistical hypothesis used in hypothesis testing, which states that there is a significant difference between the set of variables. It is often referred to as the hypothesis other than the null hypothesis, often denoted by H 1 (H-one). It is what the researcher seeks to prove in an indirect way, by using the test. It refers to a certain value of sample statistic, e.g., x¯, s, p

The acceptance of alternative hypothesis depends on the rejection of the null hypothesis i.e. until and unless null hypothesis is rejected, an alternative hypothesis cannot be accepted.

Key Differences Between Null and Alternative Hypothesis

The important points of differences between null and alternative hypothesis are explained as under:

• A null hypothesis is a statement, in which there is no relationship between two variables. An alternative hypothesis is a statement; that is simply the inverse of the null hypothesis, i.e. there is some statistical significance between two measured phenomenon.
• A null hypothesis is what, the researcher tries to disprove whereas an alternative hypothesis is what the researcher wants to prove.
• A null hypothesis represents, no observed effect whereas an alternative hypothesis reflects, some observed effect.
• If the null hypothesis is accepted, no changes will be made in the opinions or actions. Conversely, if the alternative hypothesis is accepted, it will result in the changes in the opinions or actions.
• As null hypothesis refers to population parameter, the testing is indirect and implicit. On the other hand, the alternative hypothesis indicates sample statistic, wherein, the testing is direct and explicit.
• A null hypothesis is labelled as H 0 (H-zero) while an alternative hypothesis is represented by H 1 (H-one).
• The mathematical formulation of a null hypothesis is an equal sign but for an alternative hypothesis is not equal to sign.
• In null hypothesis, the observations are the outcome of chance whereas, in the case of the alternative hypothesis, the observations are an outcome of real effect.

There are two outcomes of a statistical test, i.e. first, a null hypothesis is rejected and alternative hypothesis is accepted, second, null hypothesis is accepted, on the basis of the evidence. In simple terms, a null hypothesis is just opposite of alternative hypothesis.

You Might Also Like:

Zipporah Thuo says

February 22, 2018 at 6:06 pm

The comparisons between the two hypothesis i.e Null hypothesis and the Alternative hypothesis are the best.Thank you.

Getu Gamo says

March 4, 2019 at 3:42 am

Thank you so much for the detail explanation on two hypotheses. Now I understood both very well, including their differences.

Jyoti Bhardwaj says

May 28, 2019 at 6:26 am

Thanks, Surbhi! Appreciate the clarity and precision of this content.

January 9, 2020 at 6:16 am

John Jenstad says

July 20, 2020 at 2:52 am

Thanks very much, Surbhi, for your clear explanation!!

Navita says

July 2, 2021 at 11:48 am

Thanks for the Comparison chart! it clears much of my doubt.

GURU UPPALA says

July 21, 2022 at 8:36 pm

Thanks for the Comparison chart!

Enock kipkoech says

September 22, 2022 at 1:57 pm

What are the examples of null hypothesis and substantive hypothesis

Leave a Reply Cancel reply

Your email address will not be published. Required fields are marked *

Save my name, email, and website in this browser for the next time I comment.

9.1 Null and Alternative Hypotheses

The actual test begins by considering two hypotheses . They are called the null hypothesis and the alternative hypothesis . These hypotheses contain opposing viewpoints.

H 0 , the — null hypothesis: a statement of no difference between sample means or proportions or no difference between a sample mean or proportion and a population mean or proportion. In other words, the difference equals 0.

H a —, the alternative hypothesis: a claim about the population that is contradictory to H 0 and what we conclude when we reject H 0 .

Since the null and alternative hypotheses are contradictory, you must examine evidence to decide if you have enough evidence to reject the null hypothesis or not. The evidence is in the form of sample data.

After you have determined which hypothesis the sample supports, you make a decision. There are two options for a decision. They are reject H 0 if the sample information favors the alternative hypothesis or do not reject H 0 or decline to reject H 0 if the sample information is insufficient to reject the null hypothesis.

Mathematical Symbols Used in H 0 and H a :

H 0 always has a symbol with an equal in it. H a never has a symbol with an equal in it. The choice of symbol depends on the wording of the hypothesis test. However, be aware that many researchers use = in the null hypothesis, even with > or < as the symbol in the alternative hypothesis. This practice is acceptable because we only make the decision to reject or not reject the null hypothesis.

Example 9.1

H 0 : No more than 30 percent of the registered voters in Santa Clara County voted in the primary election. p ≤ 30 H a : More than 30 percent of the registered voters in Santa Clara County voted in the primary election. p > 30

A medical trial is conducted to test whether or not a new medicine reduces cholesterol by 25 percent. State the null and alternative hypotheses.

Example 9.2

We want to test whether the mean GPA of students in American colleges is different from 2.0 (out of 4.0). The null and alternative hypotheses are the following: H 0 : μ = 2.0 H a : μ ≠ 2.0

We want to test whether the mean height of eighth graders is 66 inches. State the null and alternative hypotheses. Fill in the correct symbol (=, ≠, ≥, <, ≤, >) for the null and alternative hypotheses.

• H 0 : μ __ 66
• H a : μ __ 66

Example 9.3

We want to test if college students take fewer than five years to graduate from college, on the average. The null and alternative hypotheses are the following: H 0 : μ ≥ 5 H a : μ < 5

We want to test if it takes fewer than 45 minutes to teach a lesson plan. State the null and alternative hypotheses. Fill in the correct symbol ( =, ≠, ≥, <, ≤, >) for the null and alternative hypotheses.

• H 0 : μ __ 45
• H a : μ __ 45

Example 9.4

An article on school standards stated that about half of all students in France, Germany, and Israel take advanced placement exams and a third of the students pass. The same article stated that 6.6 percent of U.S. students take advanced placement exams and 4.4 percent pass. Test if the percentage of U.S. students who take advanced placement exams is more than 6.6 percent. State the null and alternative hypotheses. H 0 : p ≤ 0.066 H a : p > 0.066

On a state driver’s test, about 40 percent pass the test on the first try. We want to test if more than 40 percent pass on the first try. Fill in the correct symbol (=, ≠, ≥, <, ≤, >) for the null and alternative hypotheses.

• H 0 : p __ 0.40
• H a : p __ 0.40

Collaborative Exercise

Bring to class a newspaper, some news magazines, and some internet articles. In groups, find articles from which your group can write null and alternative hypotheses. Discuss your hypotheses with the rest of the class.

This book may not be used in the training of large language models or otherwise be ingested into large language models or generative AI offerings without OpenStax's permission.

Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute Texas Education Agency (TEA). The original material is available at: https://www.texasgateway.org/book/tea-statistics . Changes were made to the original material, including updates to art, structure, and other content updates.

Access for free at https://openstax.org/books/statistics/pages/1-introduction

• Authors: Barbara Illowsky, Susan Dean
• Publisher/website: OpenStax
• Book title: Statistics
• Publication date: Mar 27, 2020
• Location: Houston, Texas
• Book URL: https://openstax.org/books/statistics/pages/1-introduction
• Section URL: https://openstax.org/books/statistics/pages/9-1-null-and-alternative-hypotheses

© Apr 16, 2024 Texas Education Agency (TEA). The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.

If you could change one thing about college, what would it be?

Graduate faster

Better quality online classes

Flexible schedule

Access to top-rated instructors

Null vs. Alternative Hypothesis

04.28.2023 • 5 min read

Sarah Thomas

Subject Matter Expert

Learn about a null versus alternative hypothesis and what they show with examples for each. Also go over the main differences and similarities between them.

In This Article

What Is a Null Hypothesis?

What is an alternative hypothesis, outcomes of a hypothesis test.

Main Differences Between Null & Alternative Hypothesis

Similarities Between Null & Alternative Hypothesis

Hypothesis Testing & Errors

In statistics, you’ll draw insights or “inferences” about population parameters using data from a sample. This process is called inferential statistics.

To make statistical inferences, you need to determine if you have enough evidence to support a certain hypothesis about the population. This is where null and alternative hypotheses come into play!

In this article, we’ll explain the differences between these two types of hypotheses, and we’ll explain the role they play in hypothesis testing.

Imagine you want to know what percent of Americans are vegetarians. You find a Gallup poll claiming ‌5% of the population was vegetarian in 2018, but your intuition tells you vegetarianism is on the rise and that ‌far more than 5% of Americans are vegetarian today.

To investigate further, you collect your own sample data by surveying 1,000 randomly selected Americans. You’ll use this random sample to determine whether it’s likely ‌the true population proportion of vegetarians is, in fact, 5% (as the Gallup data suggests) or whether it could be the case that the percentage of vegetarians is now higher.

Notice ‌that your investigation involves two rival hypotheses about the population. One hypothesis is that the proportion of vegetarians is 5%. The other hypothesis is that the proportion of vegetarians is greater than 5%. In statistics, we would call the first hypothesis the null hypothesis, and the second hypothesis the alternative hypothesis. The null hypothesis ( H 0 H_0 H 0 ​ ) represents the status quo or what is assumed to be true about the population at the start of your investigation.

Null Hypothesis

In hypothesis testing, the null hypothesis ( H 0 H_0 H 0 ​ ) is the default hypothesis.

It's what the status quo assumes to be true about the population.

The alternative hypothesis ( H a H_a H a ​ or H 1 H_1 H 1 ​ ) is the hypothesis that stands contrary to the null hypothesis. The alternative hypothesis ‌represents the research hypothesis—what you as the statistician are trying to prove with your data .

In medical studies, where scientists are trying to demonstrate whether a treatment has a significant effect on patient outcomes, the alternative hypothesis represents the hypothesis that the treatment does have an effect, while the null hypothesis represents the assumption that the treatment has no effect.

Alternative Hypothesis

The alternative hypothesis ( H a H_a H a ​ or H 1 H_1 H 1 ​ ) is the hypothesis being proposed in opposition to the null hypothesis.

Examples of Null and Alternative Hypotheses

In a hypothesis test, the null and alternative hypotheses must be mutually exclusive statements, meaning both hypotheses cannot be true at the same time. For example, if the null hypothesis includes an equal sign, the alternative hypothesis must state that the values being mentioned are “not equal” in some way.

Your hypotheses will also depend on the formulation of your test—are you running a one-sample T-test, a two-sample T-test, F-test for ANOVA , or a Chi-squared test? It also matters whether you are conducting a directional one-tailed test or a nondirectional two-tailed test.

Example 1: Two-Tailed T-test

Null Hypothesis: The population mean is equal to some number, x. 𝝁 = x

Alternative Hypothesis: The population mean is not equal to x. 𝝁 ≠ x

Example 2: One-tailed T-test (Right-Tailed)

Null Hypothesis: The population mean is less than or equal to some number, x. 𝝁 ≤ x Alternative Hypothesis: The population mean is greater than x. 𝝁 > x

Example 3: One-tailed T-test (Left-Tailed)

Null Hypothesis: The population mean is greater than or equal to some number, x. 𝝁 ≥ x

Alternative Hypothesis: The population mean is less than x. 𝝁 < x

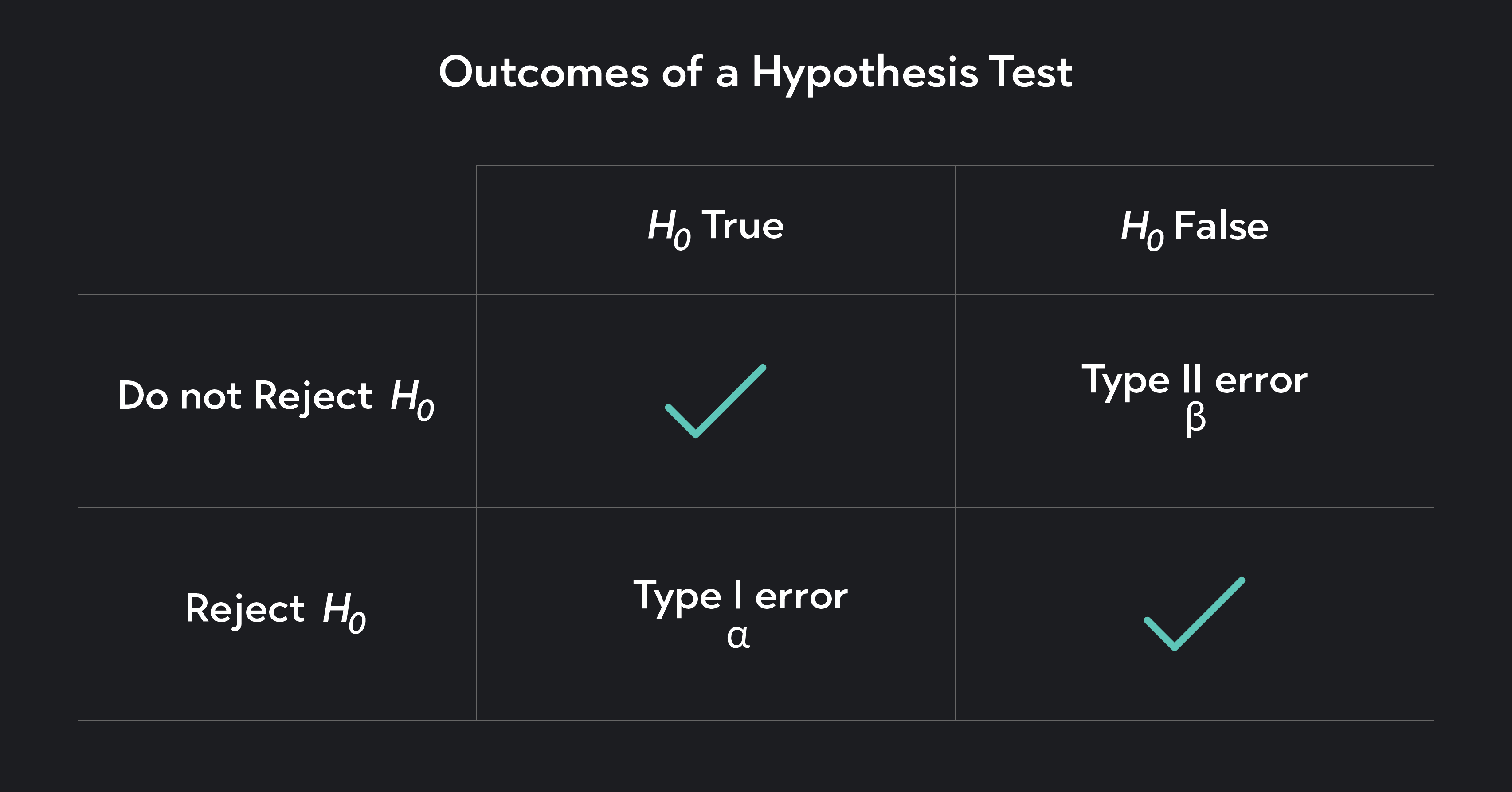
By the end of a hypothesis test, you will have reached one of two conclusions.

You will run into either 2 outcomes:

Fail to reject the null hypothesis on the grounds that there's insufficient evidence to move away from the null hypothesis

Reject the null hypothesis in favor of the alternative.

If you’re ‌confused about the outcomes of a hypothesis test, a good analogy is a jury trial. In a jury trial, the defendant is innocent until proven guilty. To reach a verdict of guilt, the jury must find strong evidence (beyond a reasonable doubt) that the defendant committed the crime.

This is analogous to a statistician who must assume the null hypothesis is true unless they can uncover strong evidence ( a p-value less than or equal to the significance level) in support of the alternative hypothesis.

Notice also, that a jury never concludes a defendant is innocent—only that the defendant is guilty or not guilty. This is similar to how we never conclude that the null hypothesis is true. In a hypothesis test, we never conclude ‌that the null hypothesis is true. We can only “reject” the null hypothesis or “fail to reject” it.

In this video, let’s look at the jury example again, the reasoning behind hypothesis testing, and how to form a test. It starts by stating your null and alternative hypotheses.

Main Differences Between Null and Alternative Hypothesis

Here is a summary of the key differences between the null and the alternative hypothesis test.

The null hypothesis represents the status quo; the alternative hypothesis represents an alternative statement about the population.

The null and the alternative are mutually exclusive statements, meaning both statements cannot be true at the same time.

In a medical study, the null hypothesis represents the assumption that a treatment has no statistically significant effect on the outcome being studied. The alternative hypothesis represents the belief that the treatment does have an effect.

The null hypothesis is denoted by H_0 ; the alternative hypothesis is denoted by H_a H_1

You “fail to reject” the null hypothesis when the p-value is larger than the significance level. You “reject” the null hypothesis in favor of the alternative hypothesis when the p-value is less than or equal to your test’s significance level.

Similarities Between Null and Alternative Hypothesis

The similarities between the null and alternative hypotheses are as follows.

Both the null and the alternative are statements about the same underlying data.

Both statements provide a possible answer to a statistician’s research question.

The same hypothesis test will provide evidence for or against the null and alternative hypotheses.

Hypothesis Testing and Errors

Always remember that statistical inference provides you with inferences based on probability rather than hard truths. Anytime you conduct a hypothesis test, there is a chance that you’ll reach the wrong conclusion about your data.

In statistics, we categorize these wrong conclusions into two types of errors:

Type I Errors

Type II Errors

Type I Error (ɑ)

A Type I error occurs when you reject the null hypothesis when, in fact, the null hypothesis is true. This is sometimes called a false positive and is analogous to a jury that falsely convicts an innocent defendant. The probability of making this type of error is represented by alpha, ɑ.

Type II Error (ꞵ)

A Type II error occurs when you fail to reject the null hypothesis when, in fact, the null hypothesis is false. This is sometimes called a false negative and is analogous to a jury that reaches a verdict of “not guilty,” when, in fact, the defendant has committed the crime. The probability of making this type of error is represented by beta, ꞵ.

Explore Outlier's Award-Winning For-Credit Courses

Outlier (from the co-founder of MasterClass) has brought together some of the world's best instructors, game designers, and filmmakers to create the future of online college.

Check out these related courses:

Intro to Statistics

How data describes our world.

Intro to Microeconomics

Why small choices have big impact.

Intro to Macroeconomics

How money moves our world.

Intro to Psychology

The science of the mind.

Related Articles

Degrees of Freedom In Statistics

Explore degrees of freedom. Learn about their importance, calculation methods, and two test types. Plus dive into solved examples for better understanding.

What Is Standard Error? Statistics Calculation and Overview

Learn what is standard error in statistics. This overview explains the definition, the process, the difference with standard deviation, and includes examples.

What Are Quartiles? Statistics 101

Learn what quartiles are and how they work in statistics. Understand how to calculate them and why even learn them.

Further Reading

What is the second derivative test [full guide], how to find derivatives in 3 steps, test statistics: definition, formulas & examples, what is the product rule [with examples], understanding math probability - definition, formula & how to find it, what is a residual in stats.

• Science, Tech, Math ›
• Statistics ›
• Inferential Statistics ›

Null Hypothesis and Alternative Hypothesis

• Inferential Statistics
• Statistics Tutorials
• Probability & Games
• Descriptive Statistics
• Applications Of Statistics
• Math Tutorials
• Pre Algebra & Algebra
• Exponential Decay
• Worksheets By Grade
• Ph.D., Mathematics, Purdue University
• M.S., Mathematics, Purdue University
• B.A., Mathematics, Physics, and Chemistry, Anderson University

Hypothesis testing involves the careful construction of two statements: the null hypothesis and the alternative hypothesis. These hypotheses can look very similar but are actually different.

How do we know which hypothesis is the null and which one is the alternative? We will see that there are a few ways to tell the difference.

The Null Hypothesis

The null hypothesis reflects that there will be no observed effect in our experiment. In a mathematical formulation of the null hypothesis, there will typically be an equal sign. This hypothesis is denoted by H 0 .

The null hypothesis is what we attempt to find evidence against in our hypothesis test. We hope to obtain a small enough p-value that it is lower than our level of significance alpha and we are justified in rejecting the null hypothesis. If our p-value is greater than alpha, then we fail to reject the null hypothesis.

If the null hypothesis is not rejected, then we must be careful to say what this means. The thinking on this is similar to a legal verdict. Just because a person has been declared "not guilty", it does not mean that he is innocent. In the same way, just because we failed to reject a null hypothesis it does not mean that the statement is true.

For example, we may want to investigate the claim that despite what convention has told us, the mean adult body temperature is not the accepted value of 98.6 degrees Fahrenheit . The null hypothesis for an experiment to investigate this is “The mean adult body temperature for healthy individuals is 98.6 degrees Fahrenheit.” If we fail to reject the null hypothesis, then our working hypothesis remains that the average adult who is healthy has a temperature of 98.6 degrees. We do not prove that this is true.

If we are studying a new treatment, the null hypothesis is that our treatment will not change our subjects in any meaningful way. In other words, the treatment will not produce any effect in our subjects.

The Alternative Hypothesis

The alternative or experimental hypothesis reflects that there will be an observed effect for our experiment. In a mathematical formulation of the alternative hypothesis, there will typically be an inequality, or not equal to symbol. This hypothesis is denoted by either H a or by H 1 .

The alternative hypothesis is what we are attempting to demonstrate in an indirect way by the use of our hypothesis test. If the null hypothesis is rejected, then we accept the alternative hypothesis. If the null hypothesis is not rejected, then we do not accept the alternative hypothesis. Going back to the above example of mean human body temperature, the alternative hypothesis is “The average adult human body temperature is not 98.6 degrees Fahrenheit.”

If we are studying a new treatment, then the alternative hypothesis is that our treatment does, in fact, change our subjects in a meaningful and measurable way.

The following set of negations may help when you are forming your null and alternative hypotheses. Most technical papers rely on just the first formulation, even though you may see some of the others in a statistics textbook.

• Null hypothesis: “ x is equal to y .” Alternative hypothesis “ x is not equal to y .”
• Null hypothesis: “ x is at least y .” Alternative hypothesis “ x is less than y .”
• Null hypothesis: “ x is at most y .” Alternative hypothesis “ x is greater than y .”
• What 'Fail to Reject' Means in a Hypothesis Test
• Type I and Type II Errors in Statistics
• An Example of a Hypothesis Test
• The Runs Test for Random Sequences
• An Example of Chi-Square Test for a Multinomial Experiment
• The Difference Between Type I and Type II Errors in Hypothesis Testing
• What Level of Alpha Determines Statistical Significance?
• What Is the Difference Between Alpha and P-Values?
• What Is ANOVA?
• How to Find Critical Values with a Chi-Square Table
• Example of a Permutation Test
• Degrees of Freedom for Independence of Variables in Two-Way Table
• Example of an ANOVA Calculation
• How to Find Degrees of Freedom in Statistics
• How to Construct a Confidence Interval for a Population Proportion
• Degrees of Freedom in Statistics and Mathematics

User Preferences

Content preview.

Arcu felis bibendum ut tristique et egestas quis:

• Ut enim ad minim veniam, quis nostrud exercitation ullamco laboris
• Duis aute irure dolor in reprehenderit in voluptate
• Excepteur sint occaecat cupidatat non proident

Keyboard Shortcuts

6a.1 - introduction to hypothesis testing, basic terms section  .

The first step in hypothesis testing is to set up two competing hypotheses. The hypotheses are the most important aspect. If the hypotheses are incorrect, your conclusion will also be incorrect.

The two hypotheses are named the null hypothesis and the alternative hypothesis.

The goal of hypothesis testing is to see if there is enough evidence against the null hypothesis. In other words, to see if there is enough evidence to reject the null hypothesis. If there is not enough evidence, then we fail to reject the null hypothesis.

Consider the following example where we set up these hypotheses.

Example 6-1 Section  

A man, Mr. Orangejuice, goes to trial and is tried for the murder of his ex-wife. He is either guilty or innocent. Set up the null and alternative hypotheses for this example.

Putting this in a hypothesis testing framework, the hypotheses being tested are:

• The man is guilty
• The man is innocent

Let's set up the null and alternative hypotheses.

\(H_0\colon \) Mr. Orangejuice is innocent

\(H_a\colon \) Mr. Orangejuice is guilty

Remember that we assume the null hypothesis is true and try to see if we have evidence against the null. Therefore, it makes sense in this example to assume the man is innocent and test to see if there is evidence that he is guilty.

The Logic of Hypothesis Testing Section  

We want to know the answer to a research question. We determine our null and alternative hypotheses. Now it is time to make a decision.

The decision is either going to be...

• reject the null hypothesis or...
• fail to reject the null hypothesis.

Consider the following table. The table shows the decision/conclusion of the hypothesis test and the unknown "reality", or truth. We do not know if the null is true or if it is false. If the null is false and we reject it, then we made the correct decision. If the null hypothesis is true and we fail to reject it, then we made the correct decision.

So what happens when we do not make the correct decision?

When doing hypothesis testing, two types of mistakes may be made and we call them Type I error and Type II error. If we reject the null hypothesis when it is true, then we made a type I error. If the null hypothesis is false and we failed to reject it, we made another error called a Type II error.

Types of errors

The “reality”, or truth, about the null hypothesis is unknown and therefore we do not know if we have made the correct decision or if we committed an error. We can, however, define the likelihood of these events.

\(\alpha\) and \(\beta\) are probabilities of committing an error so we want these values to be low. However, we cannot decrease both. As \(\alpha\) decreases, \(\beta\) increases.

Example 6-1 Cont'd... Section  

A man, Mr. Orangejuice, goes to trial and is tried for the murder of his ex-wife. He is either guilty or not guilty. We found before that...

• \( H_0\colon \) Mr. Orangejuice is innocent
• \( H_a\colon \) Mr. Orangejuice is guilty

Interpret Type I error, \(\alpha \), Type II error, \(\beta \).

As you can see here, the Type I error (putting an innocent man in jail) is the more serious error. Ethically, it is more serious to put an innocent man in jail than to let a guilty man go free. So to minimize the probability of a type I error we would choose a smaller significance level.

Try it! Section  

An inspector has to choose between certifying a building as safe or saying that the building is not safe. There are two hypotheses:

• Building is safe
• Building is not safe

Set up the null and alternative hypotheses. Interpret Type I and Type II error.

\( H_0\colon\) Building is not safe vs \(H_a\colon \) Building is safe

Power and \(\beta \) are complements of each other. Therefore, they have an inverse relationship, i.e. as one increases, the other decreases.